Image source

Demonstrating a mathematical concept is a great challenge for a mathematics teacher and it is also a challenge for the fulfillment of being able to generate meaningful learning through mathematical demonstration as a versatile instrument to fulfill the function of explaining a concept, and that this in turn is understood as that educational approach that carries an implicit meaning, and therefore should serve as a stimulus to go beyond the slight understanding that the demonstration is supposed to leave in their students.

It is important to be able to demonstrate a law, a theorem, an axiom, since by nature the human being does not like anything imposed, therefore in order to generate a significant learning in the student it is important that there is a stimulus in which he knows which are the origins of these mathematical concepts.

When we expose an idea to demonstrate a mathematical concept, the teacher must be able to break down a set of reasons in a coherent way and that do not lack the mathematical logic that is covered by that set of colorarios and axioms that can ratify the veracity of the concepts that are given as true.

The fact that the teacher in mathematics can generate a learning with stimulus in the student has a meaning in the fact that he is explaining a concept that carries an implicit validation in the affirmation of this concept, so that the student will not learn this concept in an absolute and imposed way, but on the contrary it will end up being a concept learned with an awareness in the knowledge of the origin of the explained concept.

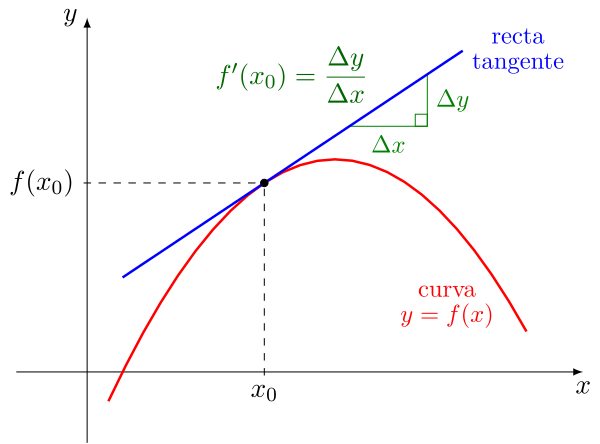
I want to take an example based on my experience as a calculus teacher, when I am explaining the derivative of a function, I tell them that geometrically the derivative of a function is the calculation of the slope of the tangent line to a function at a given point, this concept is supported by its graphical and geometric demonstration that will help to stimulate meaningful learning in my calculus students.

Image source

Once this knowledge has been assimilated, each time the student performs an exercise to find the derivative of a function, he/she will be aware that he/she is calculating the value of the slope of the tangent line to the function at a given point (x,y).